Olympiade Math – Algèbre 02 – Ex 14

Olympiade de Mathématique

( compétition de math destinée aux élèves des lycées et collèges)

Des exercices et sujets corrigés pour s’entraîner.

 

Olympiade Math – Algèbre 02 – Exercice 14
x,y et z trois réels tel que:


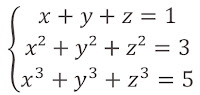
Montrer que: xyz=1/3.

Solution

* on a
x+y+z=1
(x+y+z)³=1
(x+y+z)²(x+y+z)=1
(x²+y²+z²+2xy+2xz+2yz)(x+y+z)=1
(x³+y³+z³)+(3x²y+3xy²+3x²z+3z²x+3y²z+3yz²)+6xyz=1 ①
* calculons:
A=3x²y+3xy²+3x²z+3z²x+3y²z+3yz²
A=3x²(y+z)+3y²(x+z)+3z²(x+y)
 
* on a: x+y+z=1
➝ x+y=1-z
A=3x²(1-x)+3y²(1-y)+3z²(1-z)
A=3x²+3y²+3z²-3x³-3y³-3z³
A=3(x²+y²+z²)-3(x³+y³+z³)
A=3x3-3x5= -6
①➝ 5-6+6xyz=1
➡️ xyz=2/6=1/3
 
 



1- On a
x + y = 1➝ (x + y)² = 1
➝ (x²+y²)+2xy=1
➝ 1+2xy=1
➡️ xy=0
 
2- On a
x + y + z = 1
➝ (x + y + z)² = 1
(x² + y² + z²)+2(xy+yz+zx)=1
1+2(xy+yz+zx)=1
xy+yz+zx=0 ①

* d’autre part
A=(x + y + z)(x² + y² + z²)=1
A=(x³ + y³ + z³)+(x+y+z)(xy+yz+zx)-3xyz
➝ 1+1ｘ0-3xyz=1
➡️ xyz=0

Olympiade de Maths, c’est une gymnastique de l’esprit, Ce qu’il faut c’est 4math.net et beaucoup de pratiques
4math.net Le première clé pour être bon en maths